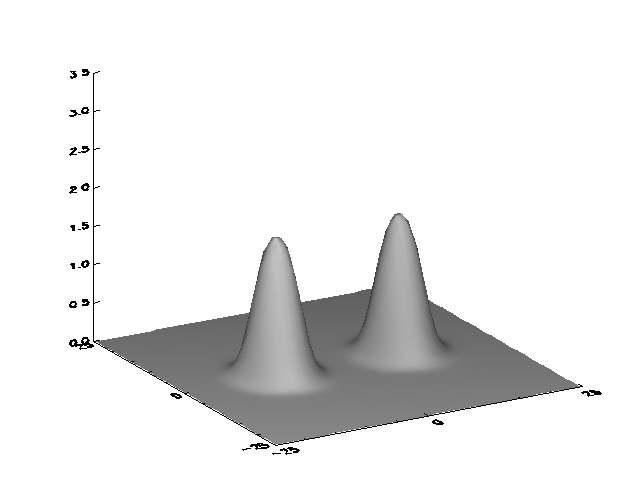
(Click on the figures to view the simulation. Notice the Partial Right Angle
Scattering)Dynamics of Qballs
Main focus: Dynamical Evolution and Interactions of Nontopological Solitons or QBalls.
Talk presented at the Meeting on Topological Defects at Capri 7-12 September 2000.
See also plots showing 3D scattering of Q balls leading to formation of Q loops
Authors: Minos Axenides, Stavros Komineas, Leandros Perivolaropoulos and Emmanuel Floratos.
Abstract:
We use numerical simulations and semi-analytical methods to investigate the
stability and the interactions of nontopological
stationary qball solutions. In the context of a simple model we map the parameter
sectors of stability for a single qball and verify the result using numerical
simulations of time evolution. The system of two interacting qballs is
also studied in one and two space dimensions.
We find that the system generically performs breather type oscillations
with frequency equal to the difference of the internal qball frequencies.
This result is shown to be consistent with the form of the qball interaction potential.
Finally we perform simulations of qball scattering and show that the right angle
scattering effect observed in topological soliton scattering in two dimensions,
persists also in the case of qballs where no topologically conserved quantities are
present. For relativistic collision velocities the qball charge is split
into a forward and a right angle scattering component. As the
collision velocity increases, the forward component gets amplified at the
expense of the right angle component.
Work available in print: PS file of the paper with figures, Source TeX File , Figures
Numerical Simulations of QBall Scattering (charge density evolution).
Identical Charge (intermediate rest frame initial velocity v=0.4)
(Click on the figures to view the simulation. Notice the Partial Right Angle
Scattering)
Zero Initial Velocity (breather-type evolution)
High Initial Velocity (v=0.8) (forward scattering)
Opposite Charge (intermediate rest frame initial velocity v=0.4)
Reviews on Non-Topological Solitons and Q Balls:
NONTOPOLOGICAL SOLITONS.
By T.D. Lee (Columbia U.), Y. Pang (Brookhaven). CU-TP-506, (Received Nov 1991). 143pp.
Published in Phys.Rept.221:251-350,1992
Q BALLS.
By Sidney Coleman (Harvard U.). HUTP-85/A050, Jun 1985. 33pp.
Published in Nucl.Phys.B262:263,1985, Erratum-ibid.B269:744,1986
Visits: